Electromagnetic waves and interference

Introduction to electromagnetic waves

Electromagnetic radiation is one of the many ways that energy travels through space. The heat from a burning fire, the light from the sun, the X-rays used by your doctor, as well as the energy used to cook food in a microwave are all forms of electromagnetic radiation. While these forms of energy might seem quite different from one another, they are related in that they all exhibit wavelike properties.

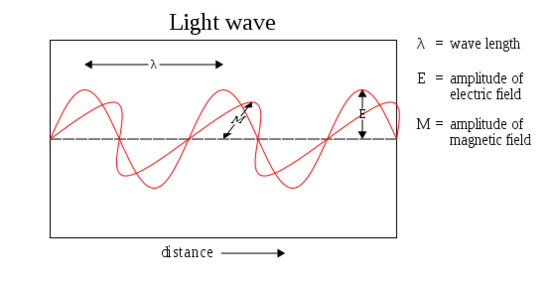
If you’ve ever gone swimming in the ocean, you are already familiar with waves. Waves are simply disturbances in a particular physical medium or a field, resulting in a vibration or oscillation. The swell of a wave in the ocean, and the subsequent dip that follows, is simply a vibration or oscillation of the water at the ocean’s surface. Electromagnetic waves are similar, but they are also distinct in that they actually consist of $2$2 waves oscillating perpendicular to one another. One of the waves is an oscillating magnetic field; the other is an oscillating electric field. This can be visualized as follows:

Electromagnetic radiation can be drawn as an oscilating electric field (oscilating in the plane of the page/computer screen), and a perpendicular (in this case, oscillating in and out of the page) magnetic field. The Y axis is amplitude, and the X axis is distance in space.

Electromagnetic waves consist of an oscillating electric field with a perpendicular oscillating magnetic field. Image from UC Davis ChemWiki, CC-BY-NC-SA 3.0

While it’s good to have a basic understanding of what electromagnetic radiation is, most chemists are less interested in the physics behind this type of energy, and are far more interested in how these waves interact with matter. More specifically, chemists study how different forms of electromagnetic radiation interact with atoms and molecules. From these interactions, a chemist can get information about a molecule’s structure, as well as the types of chemical bonds it contains. Before we talk about that, however, it’s necessary to talk a little bit more about the physical properties of light waves.

Basic properties of waves: Amplitude, wavelength, and frequency

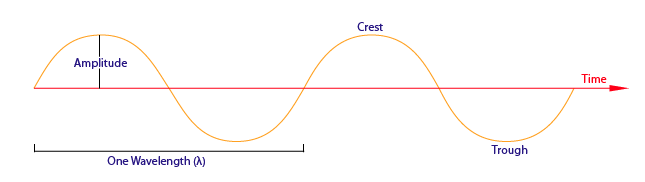
As you might already know, a wave has a trough (lowest point) and a crest (highest point). The vertical distance between the tip of a crest and the wave’s central axis is known as its amplitude. This is the property associated with the brightness, or intensity, of the wave. The horizontal distance between two consecutive troughs or crests is known as the wavelength of the wave. These lengths can be visualized as follows:

A two-dimensional representation of a wave. The amplitude is the distance from its central axis (indicated by the red line) to the tip of a crest. The wavelength is the distance from crest to crest, or from trough to trough.

The basic characteristics of a wave, including amplitude and wavelength. Image from UC Davis ChemWiki, CC-BY-NC-SA 3.0.

Keep in mind that some waves (including electromagnetic waves) also oscillate in space, and therefore they are oscillating at a given position as time passes. The quantity known as the wave’s frequency refers to the number of full wavelengths that pass by a given point in space every second; the SI unit for frequency is Hertz $(\text{Hz})$left parenthesis, start text, H, z, end text, right parenthesis, which is equivalent to “per seconds” $\Big($left parenthesiswritten as $\dfrac{1}{\text{s}}$start fraction, 1, divided by, start text, s, end text, end fraction or $\text{s}^{-1}\Big)$start text, s, end text, start superscript, minus, 1, end superscript, right parenthesis. As you might imagine, wavelength and frequency are inversely proportional: that is, the shorter the wavelength, the higher the frequency, and vice versa. This relationship is given by the following equation:

$c=\lambda \nu$c, equals, lambda, \nu

where $\lambda$lambda (the Greek lambda) is the wavelength (in meters, $\text{m}$start text, m, end text) and $\nu$\nu (the Greek nu) is the frequency (in Hertz, $\text{Hz}$start text, H, z, end text). Their product is the constant $c$c, the speed of light, which is equal to $3.00\times10^8 \text{ m/s}$3, point, 00, times, 10, start superscript, 8, end superscript, start text, space, m, slash, s, end text. This relationship reflects an important fact: all electromagnetic radiation, regardless of wavelength or frequency, travels at the speed of light.

To illustrate the relationship between frequency and wavelength, let’s consider an example.

Example: Calculating the wavelength of a light wave

A particular wave of electromagnetic radiation has a frequency of $1.5\times10^{14}\text{ Hz}$1, point, 5, times, 10, start superscript, 14, end superscript, start text, space, H, z, end text.

What is the wavelength of this wave?

We can start with our equation that relates frequency, wavelength, and the speed of light.

$c=\lambda \nu$c, equals, lambda, \nu

Next, we rearrange the equation to solve for wavelength.

$\lambda=\dfrac{c}{\nu}$lambda, equals, start fraction, c, divided by, \nu, end fraction

Lastly, we plug in our given values and solve.

$\lambda=\dfrac{3.00\times10^8\dfrac{\text{m}}{\cancel{\text{s}}}}{1.5\times10^{14}\dfrac{1}{\cancel{\text{ s}}}}=2.00\times10^{-6}\text{ m}$lambda, equals, start fraction, 3, point, 00, times, 10, start superscript, 8, end superscript, start fraction, start text, m, end text, divided by, start cancel, start text, s, end text, end cancel, end fraction, divided by, 1, point, 5, times, 10, start superscript, 14, end superscript, start fraction, 1, divided by, start cancel, start text, space, s, end text, end cancel, end fraction, end fraction, equals, 2, point, 00, times, 10, start superscript, minus, 6, end superscript, start text, space, m, end text

Concept check: What would you expect to happen to the frequency of a light wave if its wavelength were increased by a factor of $10$10?

[Show the answer]

$10$10$\dfrac{1}{10}$start fraction, 1, divided by, 10, end fraction

Period

The last quantity we will consider is the period of a wave. A wave’s period is the length of time it takes for one wavelength to pass by a given point in space. Mathematically, the period ($T$T) is simply the reciprocal of the wave’s frequency ($f$f):

$T=\dfrac{1}{f}$T, equals, start fraction, 1, divided by, f, end fraction

The units of period are seconds ($\text{s}$start text, s, end text).

Now that we have an understanding of some basic properties of waves, we’ll look at the different types of electromagnetic radiation.

The electromagnetic spectrum

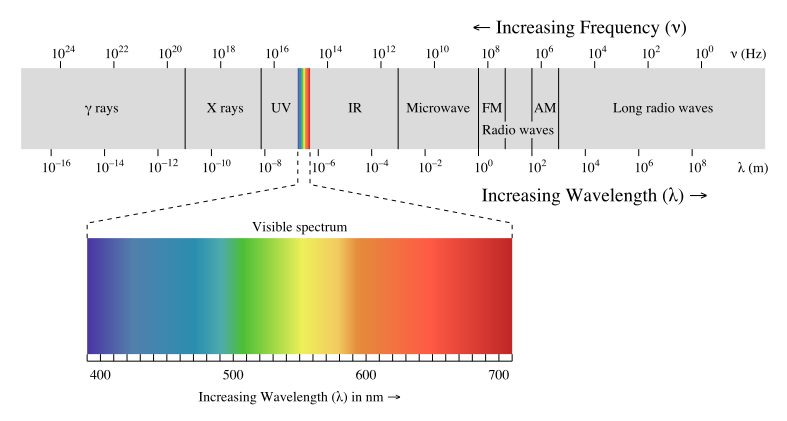
Electromagnetic waves can be classified and arranged according to their various wavelengths/frequencies; this classification is known as the electromagnetic spectrum. The following table shows us this spectrum, which consists of all the types of electromagnetic radiation that exist in our universe.

The electromagnetic spectrum is comprised of all the varieties of radiation in the universe. Gamma rays have the highest frequency, whereas radio waves have the lowest. Visible light is approximately in the middle of the spectrum, and comprises a very small fraction of the overall spectrum.

The electromagnetic spectrum. Image from UC Davis ChemWiki, CC-BY-NC-SA 3.0

As we can see, the visible spectrum—that is, light that we can see with our eyes—makes up only a small fraction of the different types of radiation that exist. To the right of the visible spectrum, we find the types of energy that are lower in frequency (and thus longer in wavelength) than visible light. These types of energy include infrared (IR) rays (heat waves given off by thermal bodies), microwaves, and radio waves. These types of radiation surround us constantly, and are not harmful, because their frequencies are so low. As we will see in the section, “the photon,” lower frequency waves are lower in energy, and thus are not dangerous to our health.

To the left of the visible spectrum, we have ultraviolet (UV) rays, X-rays, and gamma rays. These types of radiation are harmful to living organisms, due to their extremely high frequencies (and thus, high energies). It is for this reason that we wear suntan lotion at the beach (to block the UV rays from the sun) and why an X-ray technician will place a lead shield over us, in order to prevent the X-rays from penetrating anything other than the area of our body being imaged. Gamma rays, being the highest in frequency and energy, are the most damaging. Luckily though, our atmosphere absorbs gamma rays from outer space, thereby protecting us from harm.

Next, we will talk about the relationship between a wave’s frequency and its energy.

Quantization of energy and the dual nature of light

We have already described how light travels through space as a wave. This has been well-known for quite some time; in fact, the Dutch physicist Christiaan Huygens first described the wave nature of light as far back as the late seventeenth century. For about $200$200 years after Huygens, physicists assumed that light waves and matter were quite distinct from one another. According to classical physics, matter was composed of particles that had mass, and whose position in space could be known; light waves, on the other hand, were considered to have zero mass, and their position in space could not be determined. Because they were considered to be in different categories, scientists did not have a good understanding of how light and matter interacted. This all changed in $1900$1900, however, when the physicist Max Planck began studying blackbodies – bodies heated until they began to glow.

Molten lava acts as a blackbody, emitting electromagnetic radiation in the visible region at very high temperatures.

Molten lava emitting blackbody radiation. Image courtesy of the U.S. Geological Survey.

Planck found that the electromagnetic radiation emitted by blackbodies could not be explained by classical physics, which postulated that matter could absorb or emit any quantity of electromagnetic radiation. Planck observed that matter actually absorbed or emitted energy only in whole-number multiples of the value $h\nu$h, \nu, where $h$h is Planck’s constant, $6.626\times10^{-34}\text{ J}\cdot\text{s}$6, point, 626, times, 10, start superscript, minus, 34, end superscript, start text, space, J, end text, dot, start text, s, end text, and $\nu$\nu is the frequency of the light absorbed or emitted. This was a shocking discovery, because it challenged the idea that energy was continuous, and could be transferred in any amount. The reality, which Planck discovered, is that energy is not continuous but quantized—meaning that it can only be transferred in individual “packets” (or particles) of the size $h\nu$h, \nu. Each of these energy packets is known as a quantum (plural: quanta).

While this might sound confusing, we are actually already very familiar with quantized systems. The money we use daily, for example, is quantized. For instance, when you go into a store, you will not see anything on sale for a price of one dollar and two and a half cents $(\$1.025)$left parenthesis, dollar sign, 1, point, 025, right parenthesis. This is because the smallest possible monetary unit is the penny—it is impossible to transfer money in any smaller amount than this. Just as we cannot pay the cashier at the store half of a cent, energy cannot be transferred in anything less than a single quantum. We can think of quanta as being like “pennies” of electromagnetic energy—the smallest possible units by which such energy can be transferred.

Planck’s discovery that electromagnetic radiation is quantized forever changed the idea that light behaves purely as a wave. In actuality, light seemed to have both wavelike and particle-like properties.

The photon

Planck’s discoveries paved the way for the discovery of the photon. A photon is the elementary particle, or quantum, of light. As we will soon see, photons can be absorbed or emitted by atoms and molecules. When a photon is absorbed, its energy is transferred to that atom or molecule. Because energy is quantized, the photon’s entire energy is transferred (remember that we cannot transfer fractions of quanta, which are the smallest possible individual “energy packets”). The reverse of this process is also true. When an atom or molecule loses energy, it emits a photon that carries an energy exactly equal to the loss in energy of the atom or molecule. This change in energy is directly proportional to the frequency of photon emitted or absorbed. This relationship is given by Planck’s famous equation:

$E=h\nu$E, equals, h, \nu

where $E$E is the energy of the photon absorbed or emitted (given in Joules, $\text{J}$start text, J, end text), $\nu$\nu is frequency of the photon (given in Hertz, $\text{Hz}$start text, H, z, end text), and $h$h is Planck’s constant, $6.626\times10^{-34}\text{ J}\cdot\text{s}$6, point, 626, times, 10, start superscript, minus, 34, end superscript, start text, space, J, end text, dot, start text, s, end text.

Example: Calculating the energy of a photon

A photon has a frequency of $2.0\times10^{24}\text{ Hz}$2, point, 0, times, 10, start superscript, 24, end superscript, start text, space, H, z, end text.

What is the energy of this photon?

First, we can apply Planck's equation.

$E=h\nu$E, equals, h, \nu

Next, we plug in our given value for the frequency, as well as the value for Planck's constant, $h$h, and solve.

$E=(6.626\times10^{-34}\text{ J}\cdot\cancel{\text{s}})\times(2.0\times 10^{24}\cancel{\text{ s}^{-1}})=1.3\times10^{-9}\text{ J}$E, equals, left parenthesis, 6, point, 626, times, 10, start superscript, minus, 34, end superscript, start text, space, J, end text, dot, start cancel, start text, s, end text, end cancel, right parenthesis, times, left parenthesis, 2, point, 0, times, 10, start superscript, 24, end superscript, start cancel, start text, space, s, end text, start superscript, minus, 1, end superscript, end cancel, right parenthesis, equals, 1, point, 3, times, 10, start superscript, minus, 9, end superscript, start text, space, J, end text

Concept check: The wavelength of orange light is about $590-635\text{ nm}$590, minus, 635, start text, space, n, m, end text, and the wavelength of green light is about $520-560\text{ nm}$520, minus, 560, start text, space, n, m, end text. Which color of light is more energetic, orange or green?

(Hint: Keep in mind what you have already learned about the relationship between wavelength and frequency.)

Conclusion

Electromagnetic radiation can be described by its amplitude (brightness), wavelength, frequency, and period. By the equation $E=h\nu$E, equals, h, \nu, we have seen how the frequency of a light wave is proportional to its energy. At the beginning of the twentieth century, the discovery that energy is quantized led to the revelation that light is not only a wave, but can also be described as a collection of particles known as photons. Photons carry discrete amounts of energy called quanta. This energy can be transferred to atoms and molecules when photons are absorbed. Atoms and molecules can also lose energy by emitting photons.